Unit 1, Day 8: Scale factor of sides and scale factor of area.

Objective: Learn about the scale factor of sides and area.

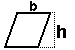
Area scale factor can apply to any shape provided you have at least there of the quantities (areas or lengths) For example here are two triangles;

Area Scale Factor 4cm-6cm

To find the area of the large triangle, you will need to first find the linear/length scale factor as the Step 1. In general to find the scale factor we divide the large quantity with the small quantity.

Scale factor = BIG/SMALL
=6/4
=1.5

Then Step 2 we would find the Area scale factor by squaring the linear scale factor;

ASF = LSF²
=1.5² = 2.25

Step 3 we would multiple the area scale factor with the small area to find the area for the large triangle.

Area = 12 x 2.25
= 27cm2

The area for the large shape is 27cm²

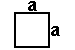
Consider a rectangle 5cm by 2cm.
The area of the rectangle is 5cm × 2cm = 10cm², but what happens if the rectangle is enlarged?
We will start by multiplying the lengths by scale factor 2.
The rectangle is now 10cm by 4cm and the area is 40 cm².
The lengths were multiplied by 2, but the area has been multiplied by a scale factor of 4.
Now we will multiply the lengths in the original rectangle by scale factor 3.
The rectangle is now 15cm by 6cm and the area is 90 cm².
The lengths were multiplied by 3, but the area has been multiplied by a scale factor of 9.
Finally, we will try multiplying the lengths by scale factor 5.
The rectangle is now 25cm by 10cm and the area is 250 cm².
The lengths were multiplied by 5, but the area has been multiplied by a scale factor of 25.
You should see a pattern! When all the lengths are multiplied by k, the areas are multiplied by k².


Questions:

1.) A hexagon has area 60 cm².
What will the area of the hexagon be, if it is enlarged with scale factor 3?

2.) Two similar rectangles are shown below:

(a) Calculate the area of rectangle A  (b) Calculate the area of rectangle B

  (c) What scale factor produces the enlargement from A to B?
  (d) How many times bigger than the area of A is the area of B?

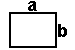
3.) A rectangle is shown below:

(a) Scale Factor of 2: (b) Scale factor of 10:

Answers:

1.) 540 cm squared (because the area scale is three squared which is nine)

2.) 
(a) 2•6=12 cm squared
(b) 24•8=192 cm squared
(c) scale factor of 1:4
(d) 16 times bigger

3.) (a) scale factor of 2= 48
     (b) scale factor of 10= 1200

Unit 1, Day 7: Similar Triangles

Objective: Learn about similar triangles.

Two triangles are similar if the only distance is size or it is flipped or turned.

All of these triangles are similar. You can see this because of the same angles and that it is just different sizes.

How to tell if two triangles are similar.

If the two triangles have to same angles. 

If the corresponding sides have the same ratio.

In similar triangles, the sides facing the equal angles are always in the same ratio.

For example: 

Triangles R and S are similar. The equal angles are marked with the same numbers of arcs.

What are the corresponding lengths?

• The lengths 7 and a are corresponding (they face the angle marked with one arc)
• The lengths 8 and 6.4 are corresponding (they face the angle marked with two arcs)
• The lengths 6 and b are corresponding (they face the angle marked with three arcs)

Calculating the Lengths of Corresponding Sides

It may be possible to calculate lengths we don't know yet. We need to:

• Step 1: Find the ratio of corresponding sides in pairs of similar triangles.
• Step 2: Use that ratio to find the unknown lengths.

Example: Find lengths a and b of Triangle S above.

Step 1: Find the ratio

We know all the sides in Triangle R, and 
We know the side 6.4 in Triangle S

The 6.4 faces the angle marked with two arcs as does the side of length 8 in triangle R.

So we can match 6.4 with 8, and so the ratio of sides in triangle S to triangle R is:

6.4 to 8

Now we know that the lengths of sides in triangle S are all 6.4/8 times the lengths of sides in triangle R.

Step 2: Use the ratio

a faces the angle with one arc as does the side of length 7 in triangle R.

a = (6.4/8) × 7 = 5.6

b faces the angle with three arcs as does the side of length 6 in triangle R.

b = (6.4/8) × 6 = 4.8

Questions:

1.) A research team wishes to determine the altitude of a mountain as follows: They use a light source at L, mounted on a structure of height 2 meters, to shine a beam of light through the top of a pole P' through the top of the mountain M'. The height of the pole is 20 meters. The distance between the altitude of the mountain and the pole is 1000 meters. The distance between the pole and the laser is 10 meters. We assume that the light source mount, the pole and the altitude of the mountain are in the same plane. Find the altitude h of the mountain. 

2.) Show that the two triangles given beside are similar and calculate the lengths of sides PQand PR. 

3.) In the triangle ABC shown below, A'C' is parallel to AC. Find the length y of BC' and the length x of A'A.

Answers: 

1.) We first draw a horizontal line LM. PP' and MM' are vertical to the ground and therefore parallel to each other. Since PP' and MM' are parallel, the triangles LPP' and LMM' are similar. Hence the proportionality of the sides gives: 

1010 / 10 = (h - 2) / 18 

Solve for h to obtain 

h = 1820 meters.

2.) ∠A = ∠P and ∠B = ∠Q, ∠C = ∠R(because ∠C = 180 - ∠A - ∠B and ∠R = 180 - ∠P - ∠Q)

Therefore, the two triangles ΔABC and ΔPQR are similar.

3.) BA is a transversal that intersects the two parallel lines A'C' and AC, hence the corresponding angles BA'C' and BAC are congruent. BC is also a transversal to the two parallel lines A'C' and AC and therefore angles BC'A' and BCA are congruent. These two triangles have two congruent angles are therefore similar and the lengths of their sides are proportional. Let us separate the two triangles as shown below. 

• 
  
  
• We now use the proportionality of the lengths of the side to write equations that help in solving for x and y.
  
  (30 + x) / 30 = 22 / 14 = (y + 15) / y
  
• An equation in x may be written as follows.
  
  (30 + x) / 30 = 22 / 14
  
• Solve the above for x.
  
  420 + 14 x = 660
  
  x = 17.1 (rounded to one decimal place).
  
• An equation in y may be written as follows.
  
  22 / 14 = (y + 15) / y
  
• Solve the above for y to obtain. 
  
  y = 26.25 

Unit 1, Day 6: Similar triangles; Angle-Angle

Objective: Similar triangles; Angle-Angle

Similar triangles are triangles that have the same shape but different sizes. If they have two similar angles, then it is automatically similar. But when you have 2 triangles and they have angles of similarity but they are different sizes it would be triangles like:

These triangles below are similar. On the smaller triangle, the medium length side is missing.

Step 1: To find what the length is, make a ratio. Put the sides that are the same over each other.

Step 2: Put 3 over 6 and x over 12.

Step 3: Cross multiply. You should come out with 6x over 36.

Step 4: Divide that by 6 for each one and you should get x over 6. That means x=6.



Questions:

1.) Find the value of X and Y and also the angle of P.

2.) Prove that ABE is similar to CDE.

3.) Are the triangles below similar? Why or why not?

Answer Key:

1.) Angle P is corresponding to Angle S so that means P = 86º. To find x use the ratio 4 over 6 and x over 9. When you cross multiply, it should come out to 6x over 36. So x=6. To find why use the ratio 4 over 6 and 7 over y. When you cross multiply, it should come out to 4y over 42. That means y=10.5.

2.) Angle ABE and CDE are similar because we already know for a fact that E is similar. Also we know that C≈A since they have the congruent symbol on the angles. Since two of the angles are similar, that means the last one has to be the same too.

3.) The triangles below are similar. Angle L and V are already congruent as we can see in the picture. Use these ratios below to know that they are similar.            

            UV    9   3           VW   15   3
            -- = -- = -           -- = -- = -
            KL   12   4           LM   20   4

Unit 1 Day 5: Similar figures: What are they and finding the missing length.

Objective:  Learn about similar figures and how to find the missing length on one side.

Similar Figures: Two figures that have the same shape but are not necessarily the same size.

These two triangles above are similar triangles. The smaller one is half the size of the larger one. 

 

These two triangles are also similar but as you can see there is a side missing. To find this missing side we can use proportions. Take two of same side from each and put it into a proportion. We will use 10 and 7 from the smaller triangle and 20 and the missing value x. Put this into a proportion of 10/7 and 20/x solve and you will find that x= 14. You can also do it easy to see if the other values are half of the larger on if so then it will be easy to do the math and multiply the small number by two.

Questions:

1.) Find the missing side length.

2.) Find missing value.

3.) Find missing value.

Answers: 

1.) 6/9 = 14/x
9x14/6= 21

2.) 18/21= 6/x
21x6/18= 7

3.) 3/4 = 9/x
4x9/3= 12
5/4 = x/12
5x12/4= 15

Unit 1 Day 4: Surface area of prism, pyramid, cylinder, cone, sphere in the real life (not fake life).

Objective: Surface area of prism, pyramid, cylinder, cone, sphere in the real life (not fake life).

We use surface area when we need to paint a house on the outside. We need to know how many gallons of paint to get. If you want to be a chemist when you are older and you are mixing elements, the larger the surface area the faster it will react. Dentists also use surface area when determining the size of dental restoration. You will need to know surface area even if you never have a job. When being a parent you need to know the surface area of a present to know how much wrapping paper you need.

Questions:

1. Name three jobs that require surface area. (not examples up top)

2. Name two thing that you can see that you think needed the surface area to make.

3. How do you know when to use surface area.

Answers:

1. Anything that makes sense.

2. Houses, cars, etc.

3. When you need to buy something like paint, etc.

Unit 1, Day 3: Surface area of prism, pyramid, cylinder, cone, and sphere

Objective: Be able to find the surface area of a prism, pyramid, cylinder, cone, and sphere

Formulas:
Cube = 6s²
where s = length of the side

Prism =  2(lw + lh + wh)
where l = length, w = width, h = height

Pyramid =  area of base + area of each of the lateral faces
 
Cylinder =  2πr (r + h)
where r = radius, h = height

Cone = πr (r + s)
where r = radius, s = slant height
 
Sphere =  4πr²
where r = radius 

Questions: 

1.) Find the surface area of the triangular prism below.

2.) Find the surface area of the sphere below.


3.) Find the surface area of the cylinder below.


Answers:

1.) 4•3=12  12÷2=6  6•2=12
7•3=21 7•4=28 5•7=35    35+28+21+12=96ft squared

2.) 18•18=324  324•4=1,296  1,296•π=4,071.5units squared

3.) 2•π•6=37.69  37.69•22=829.38" squared

Unit 1, Day 2: Introduce concept of scales (dilations)

Objective: Introduction on concept of scales and review from day 1.

Scale Factor

Definition of Scale Factor

• The ratio of any two corresponding lengths in two similar geometric figures is called as Scale Factor.
• The ratio of the length of the scale drawing to the corresponding length of the actual object is called as Scale Factor.

More about Scale Factor

• A scale factor is a number used as a multiplier in scaling.
• A scale factor is used to scale shapes in 1, 2, or 3 dimensions.
• Scale factor can be found in the following scenarios:
  1. Size Transformation: In size transformation, the scale factor is the ratio of expressing the amount of magnification.
  2. Scale Drawing: In scale drawing, the scale factor is the ratio of measurement of the drawing compared to the measurement of the original figure.
  3. Comparing Two Similar Geometric Figures: The scale factor when comparing two similar geometric figures, is the ratio of lengths of the corresponding sides.

ABCD and PQRS are similar polygons. Then the scale factor of polygon ABCD to polygon PQRS is the ratio of the lengths of the corresponding sides.

• Scale factor = BC:QR = 3:8.

Solved Example on Scale Factor

Find the scale factor from the larger rectangle to the smaller rectangle, if the two rectangles are similar.

Choices: 
A. 5:1
B. 5:6
C. 6:5
D. 6:7
Correct Answer: B
Solution:
Step 1: If we multiply the length of one side of the larger rectangle by the scale factor we get the length of the corresponding side of the smaller rectangle.
Step 2: Dimension of larger rectangle × scale factor = dimension of smaller rectangle
Step 3: 24 × scale factor = 20 [Substitute the values.]
Step 4: Scale factor = 20/24 [Divide each side by 24.]
Step 5: Scale factor =  = 5:6 [Simplify.]
Therefore, scale factor from the larger rectangle to the smaller rectangle is 5:6.

Questions: 

1. Find the image of point P (1, 2) under dilation with center (0, 0) with a scale factor of 2.

A. (- 1, - 2)

B. (2, 4)

C. (- 2, 4)

D. (2, - 4)

2. A map is constructed on a scale of 10 ft to 1 in. What is the area of the land represented on the map by an oblong measuring 4 × 5 in.?

A. 4000 ft2

B. 2000 ft2

C. 50 ft2

D. 2100 ft2

3. A map is constructed on a scale of 20 yd to 1 in. What is the area of the land represented on the map by an oblong measuring 3 × 2 in.?

A. 40 yd2

B. 2400 yd2

C. 4800 yd2

D. 2500 yd2 Answers: 1. Step 1 : To find the image of a point on the coordinate plane under dilation with center as origin, multiply the coordinates with scale factor. Step 2 : Image of P (1, 2) is P ′(1 × 2, 2 × 2) Step 3 : = (2, 4) 2.Step 1 : Scale = 10 ft to 1 in. Step 2 : The actual width of the ground = 4 × 10 = 40 ft. Step 3 : The actual length of the ground = 5 × 10 = 50 ft Step 4 : So, the area of the land = 40 × 50 = 2000 ft2. 3. Step 1 : Scale = 20 yd to 1 in. Step 2 : The actual width of the ground = 3 × 20 = 60 yd. Step 3 : The actual length of the ground = 2 × 20 = 40 yd. Step 4 : So, the area of the land = 60 × 40 = 2400 yd2.

Unit 1, Day 1: Area of squares, rectangles, triangles, trapezoids, parallelograms, circles, and polygons. Define point, line, plane, line segment, ray, angle, median, altitude, perpendicular.

Objective: Area of squares, rectangles, triangles, trapezoids, parallelograms, circles, and polygons. Define point, line, plane, line segment, ray, angle, median, altitude, perpendicular.

Area:

square = a ²                                

rectangle = ab  
triangle = 0.5(b•h)

parallelogram = bh 

trapezoid = h/2 (b₁ + b₂) 

circle = pi•r ²  

polygon = (0.5)(apothem)(perimeter)

Define: 
point = a precise location or place on a plane, usually represented by a dot

line = a geometrical object that is straight, infinitely long, and infinitely thin

plane = a two-demensional surface that you place lines, points, line segments, etc.

line segment = a part of a line connected to two points with definite ends

ray = a portion of a line which starts at a point and goes off in a particular direction to infinity

angle = the amount of turn between two straight lines that have a common endpoint (the vertex)

median = a line segment joining a vertex to the midpoint on the opposite side (a triangle has 3 medians)

altitude = another word for height

perpendicular = two lines that meet together at a 90º angle

Quiz: 

1.) Find the area of a parallelogram with the base of 8.6 in. and the height of 12.9 in.

2.) Find the area of a triangle with the triangle with a height of 15.2 in. and a base of 9.7 in.

3.) Find the area of a circle with radius of 3.1 cm.

4.) Find the area of a square with the sides being 9.32 in.

5.) Find the area of a rectangle with the sides being 3.8 and 1.9 mm.

6.) Find the area of a trapezoid with the height being 3.7 cm. and base 1 being 8.4 cm. and base 2 being 1.9 cm.

7.) What would you place a line on?

8.) What is the use of a plane?

9.) When do you think you would use a line segment?

10.) Define point.

11.) Define ray.

12.) What would you use an angle for?

13.) What would be the altitude be for a pentagon having the highest point be 12 high?

14.) How many perpendicular lines does a square have?

15.) How many medians does a pentagon have?

Answers: 

1.) 8.6x12.9=110.94 in. squared

2.) 15.2x9.7÷2=73.72 in. squared

3.) 3.1•3.1•π=30.19 cm. squared

4.) 9.32x9.32=86.8624 in. squared

5.) 3.8x9.1=34.58 mm. squared

6.) 3.7/2 (8.4+1.9)= 14.5 cm. squared

7.) You would place a line on a plane.

8.) The use of a plane is to put your line and line segments on.

9.) You would use a line segment when graphing.

10.) point = a precise location or place on a plane, usually represented by a dot

11.) ray = a portion of a line which starts at a point and goes off in a particular direction to infinity

12.) You would use a angle for finding the length of the sides.

13.) The altitude would be 12 because the height is 12 and altitude is another word for height.

14.) A square has 4 perpendicular sides.

15.) A pentagon has 5 medians.

Tips From the Pros

Unit 5, Day 6: Students will be able to write an equation of a line given data points

Objective: Students will be able to write an equation of a line given data points

The formula to find the slope is y2-y1/x2-x1

So if you have 2 points like (2,6) and (4,10), then you subtract 6 from 10 to get 4. You also subtract 2 from 4 to get 2. So now your slope is 6/2 which also equals 3. So now that you know the slope is 3, use the closest point to the y-axis and put it into the equation y-y1=m(x-x1). In this case you would want to use the point (2,6). So now your equation to find the y-intercept will look like y-6=m(x-2). Since m=3, put it into the equation to equal y-6=3(x-2). Keep simplifying it until you get it to look like y=3x. Put this into the point so it should look like 6=3(2). So the equation to these 2 points is y=3x.

Questions: 

1.) Find the slope out of these 2 points: (6,14) (-3,-12)

2.) Find the y-intercept using one of the slope on one of the points

3.) Graph the equation

Answers: 

1.) Slope = -2.9

2.) Equation = y=-2.9x-3.3 

3.) 

TIPS FROM THE PROS

Unit 5: Day 5: Students will be able to graph linear inequalities.

Objective: Students will be able to graph linear inequalities.

Step 1: Simplify the inequality you're going to graph. Multiply out anything that's in parentheses, and combine numbers that are unattached to variables.
-2x² + 5x < -6(x + 1)
-2x² + 5x < -6x - 6

Step 2: Move all terms to one side so the other is zero. It will be easiest if the highest power variable is positive. Combine common terms (such as -6x and -5x).

0 < 2x² -6x - 5x - 6
0 < 2x² -11x - 6

Step 3:  Solve for the variables. Pretend that the inequality sign is an equal sign, and find all values of the variable. If necessary, solve by factoring.

0 = 2x² -11x - 6
0 = (2x + 1)(x - 6)
2x + 1 = 0, x - 6 = 0
2x = -1, x = 6
x = -1/2, x = 6

Step 4: Draw a number line including the variable solutions (in order).

Step 5: Draw a circle on the points. If the inequality symbol is "less than" (<) or "more than" (>), draw an empty circle over the variable solution(s). If it means "less than or equal to" (≤) or "more than or equal to" (≥), then fill in the circle.

In this case the equation was greater than zero, so use open circles.

Step 6: Test your solution. Take a number from each of the resulting intervals and plug it back into the inequality. If you get a true statement once solved, shade this region of the number line.

In the interval from (-∞,-1/2) we will take -1 and plug it into the original inequality.
0 < 2x² -11x - 6
0 < 2(-1)² -11(-1) - 6
0 < 2(1) + 11 - 6
0 < 7
Zero is less than 7 is correct, so shade (-∞, -1/2) on the number line.

Next, on the interval from (-1/2, 6) we will use zero.
0 < 2(0)² -11(0) - 6
0 < 0 + 0 - 6
0 < -6
Zero is not less than negative six, so do not shade (-1/2,6).

Lastly, we will take 10 from the interval (6,∞).
0 < 2(10)² - 11(10) + 6
0 < 2(100) - 110 + 6
0 < 200 - 110 + 6
0 < 96
Zero is less than 96 is correct, so shade (6,∞) as well.

Use arrows on the end of shading to indicate that the interval continues into infinity. The completed number line:

Questions:
 
1.) Graph the following inequality  y x + 3 

2.)

Which inequality is represented by the graph below?
 

Choose:

3.) Graph the following inequality:

2x + y > 4

 

 

 

 

 

 

Answers:

 

 

1.)

2.) y<-2x+1


3.)